Forschungsschwerpunkt Partielle Differentialgleichungen
Der Schwerpunkt Partielle Differentialgleichungen: Analysis, Modellierung und Numerische Simulation deckt die gesamte Breite der Forschung zu partiellen Differentialgleichungen ab. Das Spektrum umfasst funktionalanalytische Untersuchungen; qualitative und quantitative Aussagen; Aspekte der Modellierung, der optimalen Kontrolle, der Parameteridentifizierung; die Untersuchung und Entwicklung numerischer Verfahren bis hin zur Simulation ingenieur- und naturwissenschaftlicher Aufgabenstellungen auf Hochleistungsrechnern gemeinsam mit Anwendern aus Industrie und Forschungseinrichtungen.
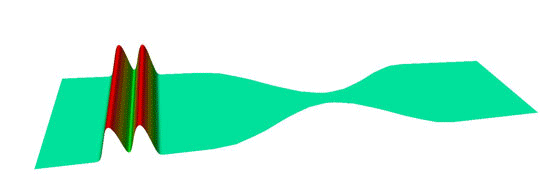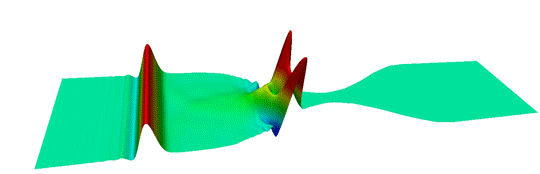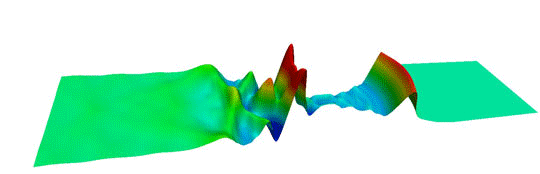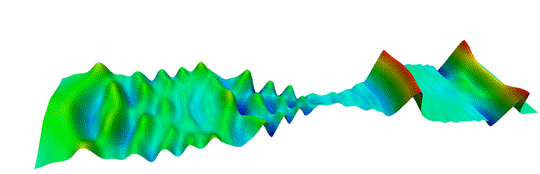
Für das KIT mit seinen ingenieur- und naturwissenschaftlichen Forschungsfeldern und -zentren wird dieser Schwerpunkt eine zentrale Rolle spielen an der Schnittstelle zwischen Grundlagenforschung und technischer Anwendung.
Das größte übergeordnete Forschungsprojekt des Schwerpunkts ist der von der DFG geförderte Sonderforschungsbereich 1173 Wellenphänomene: Analysis und Numerik, der zum 1.7.2015 am KIT eingerichtet wurde.
Vom 1.4.2006 bis 31.3.2015 förderte die DFG das Graduiertenkolleg 1294 Analysis, Simulation und Design nanotechnologischer Prozesse, an dem zehn Kollegen des Schwerpunkts beteiligt waren.
Wer über Termine und Aktivitäten des PDE-Schwerpunkts informiert werden möchte, kann sich für unseren E-Mail-Verteiler anmelden. Um Mißbrauch zu vermeiden akzeptieren wir nur E-Mail-Adressen, die wir wissenschaftlichen Einrichtungen zuordnen können.
Weitere Informationen zum Schwerpunkt
Mitglieder des PDE-Schwerpunkts
Karlsruher PDE Seminar
Karlsruher PDE Lectures
Aktivitäten des PDE Schwerpunkts
Gäste, Vorträge und Events des SFBs
